limit comparison test hard questions|9.4E: Exercises for Comparison Test

20241226 | By cache http www.wifemamafoodie.com dry-fresh-herbs-oven , DOD News

limit comparison test hard questions|9.4E: Exercises for Comparison Test : agencies In this section we will discuss using the Comparison Test and Limit Comparison . It has been revealed that ethanol-based surface disinfection can be the most effective for 3D printed bioresorbable stents (BRSs) made of polycaprolactone (PCL) [25].A .

{plog:ftitle_list}

This is an overview of proper loading of your Midmark M9 or Midmark M11 autoclave. Always refer to the appropriate technical documentation for the complete list of instructions, safety alerts, and sequence of .

The limit comparison test

Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University.10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 .Here is a set of assignement problems (for use by instructors) to accompany the .In this section we will discuss using the Comparison Test and Limit Comparison .

In this section we will discuss using the Comparison Test and Limit Comparison .The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X .

elisa test used for

This section explains the Direct and Limit Comparison Tests for determining the .Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 .The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is .Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the .

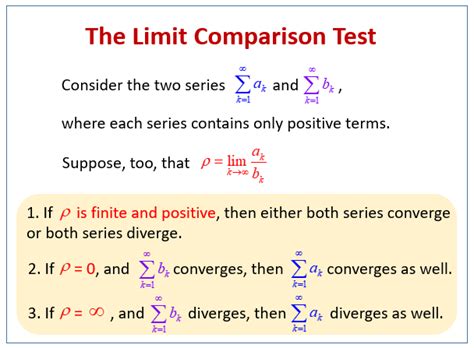
The limit comparison test

How to use the limit comparison test to determine whether or not a given series converges or .for all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n .

The limit comparison test - Ximera. We compare infinite series to each other using limits. Using . Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University. In this section we will discuss using the Comparison Test and Limit Comparison Tests to determine if an infinite series converges or diverges. In order to use either test the terms of the infinite series must be positive. Proofs for both tests are also given.The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X an and X bn either both converge or both diverge. ∞. 1. Example 1: Determine whether the series. converges or diverges. 2n + n.

This section explains the Direct and Limit Comparison Tests for determining the convergence or divergence of series. The Direct Comparison Test involves comparing terms with a known series, while the .

Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 27) ∞ ∑ n = 1(1 − 1 n)n. n (Hint: (1 − 1 n)n → 1 / e.)

The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is bn series P an and P bn either both converge or both diverge. nite and c > 0, then the two. owing series can be proven to converge or diverge by comparing to a kno.Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the convergence or divergence of series with positive terms. Discuss the limitations and advantages of each test, providing insights into their practical implications.How to use the limit comparison test to determine whether or not a given series converges or diverges, examples and step by step solutions, A series of free online calculus lectures in videos

The Limit Comparison Test (examples, solutions, videos)

for all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n = 2 1 (n2 − 1), instead we show how we can use the limit comparison test to compare. ∞ ∑ n = 2 1 n2 − 1 and ∞ ∑ n = 2 1 n2. Let us examine the idea behind the limit comparison test.The limit comparison test - Ximera. We compare infinite series to each other using limits. Using the comparison test can be hard, because finding the right sequence of inequalities is difficult. The limit comparison test eliminates this part of the method.

Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University. In this section we will discuss using the Comparison Test and Limit Comparison Tests to determine if an infinite series converges or diverges. In order to use either test the terms of the infinite series must be positive. Proofs for both tests are also given.The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X an and X bn either both converge or both diverge. ∞. 1. Example 1: Determine whether the series. converges or diverges. 2n + n. This section explains the Direct and Limit Comparison Tests for determining the convergence or divergence of series. The Direct Comparison Test involves comparing terms with a known series, while the .

Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 27) ∞ ∑ n = 1(1 − 1 n)n. n (Hint: (1 − 1 n)n → 1 / e.)The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is bn series P an and P bn either both converge or both diverge. nite and c > 0, then the two. owing series can be proven to converge or diverge by comparing to a kno.

Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the convergence or divergence of series with positive terms. Discuss the limitations and advantages of each test, providing insights into their practical implications.

How to use the limit comparison test to determine whether or not a given series converges or diverges, examples and step by step solutions, A series of free online calculus lectures in videosfor all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n = 2 1 (n2 − 1), instead we show how we can use the limit comparison test to compare. ∞ ∑ n = 2 1 n2 − 1 and ∞ ∑ n = 2 1 n2. Let us examine the idea behind the limit comparison test.

Math 2300: Calculus II Comparison Test Practice The